What is Voltammetry? Types and Applications

Voltammetry is the study of the current response of a chemical under an applied potential difference using a potentiostat. Voltammetry encompasses a number of different methods, each of which can tell us about the kinetics and thermodynamics of electron addition (reduction) and electron loss (oxidation). In addition, voltammetry can be used to test for the presence of an electroactive substance.

The applications of voltammetric techniques can be grouped into two types: sweep types, and the loosely called polarographic types (which is herein also used to refer to some non-polarographic methods). Sweep types are techniques where the solution is not stirred after each set potential, whereas polarographic types are techniques where the solution is stirred after each set potential. A separate group of methods (see "addition techniques") modify these techniques by, for example, applying an alternating current or rotating an electrode.

Sweep Type Methods

---

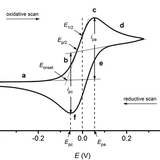
Cyclic Voltammetry

In cyclic voltammetry, potential difference is varied at a constant rate with time, between two potentials. The direction of the potential change is swapped when either of the limiting potentials is reached. The starting potential may be any potential between the two, but is often 0 V.

The main advantages of cyclic voltammetry over other voltammetric methods is the ease in interpretation of results, as well as how large potential ranges can be examined rapidly. The reversibility of oxidation and reduction, and the approximate formal potential can be readily extracted from a compound’s cyclic voltammogram.

Cyclic voltammetry can be used to determine (or measure) a number of electrochemical properties about a material, including:

• The reversibility of a reaction
• The formal reduction potential of a species
• Electron transfer kinetics
• Energy levels of semiconducting polymers

Linear Sweep Voltammetry¹

Linear sweep voltammetry is a simplification of the cyclic voltammetry experiment, where only a single linear sweep is run. Using linear sweep voltammetry, several key parameters can be observed and used diagnostically to determine thermodynamic reversibility. These are namely the peak current, the potential at the peak current, and the potential at half the peak current prior to the peak being reached.

Linear sweep voltammetry can be used to determine thermodynamic reversibility based on:

• The peak current
• The peak current potential
• The half-peak current potential

Staircase Voltammetry¹

Staircase voltammetry is where an electrode is kept at a constant potential and the current is recorded after a delay. The potential is then changed, and the current is recorded again. The step in potential and the recording of current is repeated over a given potential range. The potential change is linear, in size and direction, forming a staircase.

The method was designed for use with polarographic electrodes, but can also be used with more standard, non-polarographic ones. For a static mercury drop electrode, the mercury drop is generated, the flow of mercury stopped, and then the drop kept for the entire run of applied potential steps. After the run of potential steps, the mercury is dropped, refreshing the solution for another scan. In non-polarographic electrodes, the solution can be left for a period, or stirred to regain its initial concentration profile.

The results of this technique are similar to linear sweep voltammetry, and several staircases can be combined to create an analogue for cyclic voltammetry. The purpose of this technique is to do cyclic voltammetry / linear sweep voltammetry with suppressed charging currents. This technique, however, has not found wide application. In order to reduce the charging currents sufficiently, the sampling rate and scan rate must be low, preventing fast scans. In addition, the method has poor signal to noise.

Polarography Like Methods

---

Polarography is a very early form of voltammetry measurement, invented in 1922 by Jaroslav Hayrovský, for which he won the 1959 Nobel prize for chemistry. The technique is defined by the use of either the dropping mercury electrode or the static mercury electrode as the working electrode. Initially the technique was developed for use with a dropping mercury electrode, but in the 1980s the static mercury electrode was developed to improve on the design. In addition, some methods have been modified to use more standard non-polarogrphic electrodes.

Polarographic electrodes are designed to form a mercury drop at a given potential. The mercury drop grows, until either the flow of mercury is stopped (static mercury electrode), or the drop falls off (dropping mercury electrode). For the static mercury electrode, the drop is often formed prior to changing the potential, and then knocked off after a result is recorded. In both cases, the fall of the mercury from the electrode effectively stirs the solution, largely reproducing the starting condition prior to the initial drop formation.

Dropping mercury electrodes have other advantages, other than being able to stir a solution with a mercury drop. It takes a very low potential to produce H2 at a mercury electrode, which allows for a more negative potential range. In addition, mercury electrodes tend to have less background currents from processes in an electrode than standard non-polarographic metal electrodes. This is due in part to the continuous reformation of the mercury in the electrode, which prevents contamination.

A disadvantage is that mercury is readily oxidised at potentials greater than 0.0 V relative to the standard cadmium electrode. The ready oxidation of mercury limits the systems that can be studied.

Polarographic methods are usually forms of pulse voltammetry. Pulse voltammetry encompasses several techniques in electrochemistry in which an electrode is quickly changed to a potential, maintained at it for some time, then swapped to another potential. This process may be repeated a number of times.

Pulse voltammetry was originally designed for polarographic electrodes, but may in fact be done at any electrode. Because modern work has moved away from dropping mercury electrodes, these methods will only be described for the static mercury drop electrode. However, the original form of polarograph, DC conventional polarography, which is not strictly pulse voltammetry, will be described for the static mercury electrode.

Conventional / DC Polarography¹

The original form of polarography is referred to as DC polarography or conventional polarography. In conventional polarography the potential is varied slowly (1–3 mV s^-1), such that each drop is approximately at constant potential. In modern practice this method is often implemented with a small change in potential (1–10 mV) at the start of each drop instead.

The same results are yielded whether the potential is continuously changed or changed at the start of each drop. In DC polarography, when applied to a dropping mercury electrode, each successive drop is characterised by an increase in the current asymptotically towards a value. Generally, the peak value is what is interpreted in a dropping mercury electrode. Interpreting static mercury drop electrode is more complex and has to be done electronically. Normal pulse polarography is usually used as an alternative method.

Measuring the Concentration of a Known Compound

At a dropping mercury electrode, the peak current can be made linear with bulk concentration under certain constraints. In order to enforce linearity with concentration, the potential must be much less than the formal potential for the reduction of the species in solution. A low potential means that at the electrode, all local oxidised species are reduced instantaneously i.e. O + ne^- → R. In practice, calculations of the concentration are done by calibrating the currents to a set of standard solution. The formula which dictates the concentration dependence is the Ilkovič equation, which may be rearranged to the following form:

Where (I)_max is the diffusion current constant, n the number of electrons in the oxidation imax the peak current, D_O is the diffusion constant for compound O in cm² s^-1, C_O^* is the bulk concentration of compound O in mol cm^-3, m is the rate of mercury addition in mg s^-1 and t is the time in the drop growth.

It should be noted that approximations are made in the formation of the Ilkovič equation, but accuracies of up to ±0.1 % are possible in the region of 0.1 – 10 mM.

Calculation of the Diffusion Current Constant / n-Value

Under similar conditions to those outlined in "Measuring the concentration of a known compound", diffusion constant or n-values can be measured. In the Ilkovič equation, the diffusion constant can be calculated if n is known, and vice versa.

In water like viscosities, the value of (I)_max is approximately 1.5 – 2.5 for 1 electron processes, 3.0-4.5 for 2 electrons, and 4.5-7.0 for 3. Notable exceptions to these approximate diffusion current constants include H⁺, OH^-, O₂, polymers, and biomolecules.

Calculating the Polarographic Half Wave Potential for Reversible Systems & n Value

The polarographic half wave potentials (E_1/2) can be obtained for the reaction O + ne- ⇌ R. The system is set up with only O in solution, and the voltage scanned. As the voltage is scanned, the current is recorded a certain time into the formation of the drop and is plotted.

For a dropping mercury electrode, the current at the point of dropping the mercury is most commonly used. When the O + ne- ⇌ R reaction is reversible, the potential at half the current wave height corresponds the polarographic half wave potential (E_1/2). The process is similar for a reduction. For a reversible system the wave slope is 2.303RT/nF, (and if the reaction is known to be reversible, this may be used to calculate the n value).

Cyclic voltammetry is, however, a safer method to determine reversibility, as the reaction is observed in both directions.

Calculating Kinetic Parameters from the Irreversible System

Here the focus will be on the dropping mercury electrode, see normal pulse voltammetry for approaches for the static mercury drop electrode. For irreversible systems, the half wave potentials shift, such the previous equation no longer applies. When the reaction is completely irreversible (O + ne- → R), the following applies instead:

Where E^0' is the formal potential, k⁰ is the standard rate constant, D_O the diffusion constant of compound O, α is the transfer coefficient (see Butler-Volmer equation), E_1/2 is the potential at half the limiting current, and t_max is the time which is sampled in the drop, i.e. for a dropping mercury electrode, the point where the mercury drops.

The following equation has been found to approximate the current dependence on applied potential (E ) before the mercury drops, at 25 °C:

Where i is the current measured at a point in time during the mercury drop i.e. usually when it is dropped, and i_d is the limiting current. This equation only applies for 0.1 < (i/i_d) > 0.94. From this equation, it may be shown that the slope of an E vs. log((i_d-i)/i) plot is given by 54.2/α mV, allowing the calculation of α. The value of |E_3/4-E_1/4 | = 51.7/α, allowing another check. In addition, the extracted value of E_1/2 allows calculation of k⁰ if α, E^0', and D^O are known.

Normal Pulse Voltammetry¹

Normal pulse voltammetry was originally developed to reduce the charging current in the dropping mercury electrode which is present in DC polarography. Conventional voltammetry also depletes redox active compounds through a long pulse at a potential where electrolysis occurs. Normal pulse voltammetry aims to reduce this effect by shortening the pulse used.

The method builds on the tast polarographic method, and is also used for non-polarographic electrodes and static mercury electrode where charging currents are reduced.

In normal pulse voltammetry, the electrode is initially held at a voltage at which minimal electrolysis occurs. Subsequently the electrode is pulsed to a higher potential for a short period (1–100ms), and the current is recorded at a point within that pulse. The current is usually sampled towards the end of the pulse.

After each pulse, the drop is either dislodged or allowed to drop, depending on if a mercury dropping electrode or static mercury electrode is used. This process is usually repeated, with increasing potential applied in each pulse.

It should be noted that this method may be referred to as normal pulse polarography, when a polarographic electrode is used. The more general name "normal pulse voltammetry" encompasses both the normal pulse polarography, and when the method is applied to a non-polarographic electrode.

When the static mercury electrode is used with normal pulse voltammetry, the drop is formed, and the flow of mercury turned off prior to the potential pulse. As such, it behaves quite similarly to a non-polarographic electrode. The difference between the static mercury electrode and a non-polarographic electrode is that the mercury is dropped after the pulse. When mercury is dropped, it stirs the solution reforming it to its prior state.

With a static mercury drop electrode, it is still necessary to have a gap between the potential change and the current detection. In the early time period, there is a charging current which decays with a time constant of R_uC_d, where R_u is the uncompensated resistance, and C_d is the differential capacitance of the double layer. R_uC_d is referred to as the cell constant and has value on the order of 10–1000 μs.

By recording current after a time gap, the charging currents can be minimised. However, even when the charging current is minimised, there still exists background currents. Background currents are from the non-ideal nature of the electrode, the electrolyte, or the purity of the system. The advantages of the static mercury electrode over the dropping mercury electrode are the elimination of charging currents, and shorter drop times, which accelerate the data gathering.

Non-polarographic electrodes may be employed to do normal pulse voltammetry, but the results are poorer. Non-polarographic electrodes do not stir the mixture via mercury drop, and as such the system must be renewed via other means, such as:

• The reduction reversing when the system is brought back to the non-pulse potential
• Diffusion renewing it
• Optionally stirring the solution with, for example, a rotating disk electrode

If the diffusion layer is renewed, the results are essentially the same as at the static mercury electrode. Background currents tend to be larger for non-polarographic electrodes, due to the electrode itself. Evidence for a lack of renewal of the diffusion layer would be the voltammogram having a distinct peak current like in cyclic/linear sweep voltammetry, instead of asymptotically approaching a value.

At both the non-polarographic and static mercury electrode, the oxidation / reduction occurring at the electrode can be adjusted to be either in the reversible / quasireversible / irreversible regions. Controlling the thermodynamic reversibility in this way requires the reaction to be chemically reversible.

How thermodynamically reversible the pulse is depends on how long after the potential change the current is sampled. For a reaction O + ne- ⇌ R, it is usually expressed in terms of parameter λ⁰, where:

Here D_x is the diffusion constant for compound x, k₀ is the standard rate constant, and the time after the pulse the current is recorded. For λ⁰>2 the system may be said to be approximately reversible, for quasirevsible between 2>λ⁰>10^-2α, and for irreversible 10^-2α>λ⁰. Here, α is the transfer coefficient.

Calculation of the Diffusion Constant/Concentration/Electrode Area from a Limiting Response

Here the case for the reaction O + ne^- ⇌ R, where the pulse is to potential significantly lower that E^0' will be considered. These conditions should be such that all O is spontaneously and irreversibly reduced to R at the electrode, and this means the following equation applies.

Where A is the area of the electrode, D_O the diffusion constant of O, C_O^* the bulk concentration of O, is the time after the pulse begins the current is recorded, i is the current, and i_d is the limiting current. This equation allows the calculation of the other parameters when the rest are known. Several measurements may be made using, for example, two known C_O^* concentration, or two known electrode areas (A), to measure this dependence.

Calculating the Polarographic Half Wave Potential and n Value

Via a method completely analogous to DC polarography, the half wave potential can be calculated. The same dependence of the formal potential is observed, and the same criteria for reversibility. The slope at the half wave potential may also be used to calculate the n value via the same method in DC polarography.

Calculating Kinetic Parameters

Similarly to in DC polarography, the kinetic parameters may be calculated in normal pulse voltammetry, with the static mercury drop electrode or a non-polarographic electrode. However, the methods used are slightly different in normal pulse voltammetry. Firstly, it should be noted that if Butler-Volmer kinetics are assumed, the forward and reverse rate constants for reaction O + ne^- ⇌ R, is:

Where k_f is the forward reaction rate i.e. O + ne^- → R, k_b the reverse reaction rate, k⁰ the standard rate constant, E the applied potential, and E^0' the formal potential. From this equation, it is clear that k⁰, n, α , and E^0' are the main parameters to understand the rate. The dependence of current on rate are given by the following equations, where O is present in bulk solution, and R absent:

Where i_d is the limiting current, i the recorded current, D_x the diffusion constant for compound x, E the applied potential, E^0' the formal potential, k_f the forward reaction rate, and the time after pulse start where the current recorded. Note: λ⁰ previously defined is λ at E^0'.

This equation can be further simplified in the irreversible case as:

For irreversible curves, there are three methods for calculating the base k0 and α parameters:

1. Measuring i/i_d at various potentials, and recording F₁(λ). From here the value of λ may be determined via fitting/ graphical methods. λ may then be used to calculate k_f, if D_O is known. k_f may be plotted using log(k_f), vs E-E^0' to provide α and k₀. Acquiring E^0' is complex, however, and may require potentiometry to determine.
2. The potential at ¾ and ¼ of the peak current allow the calculation of α. The Tomeš criteria ie. |E_3/4-E_1/4| gives the α via |E_3/4-E_1/4|=45.0/α
3. Curves may be fit to the equations listed above using a least square approach.

For quasi-reversible systems, only fitting to the above equations are appropriate.

Chronoamperometry¹

Previously, current has been sampled after a delay, however the change in current with time, may also be applied to find out fundamental electrochemical parameters. The measurement of the current with respect to time after an applied potential pulse is referred to as "chronoamperometry".

Reverse Pulse Voltammetry¹

The pulse scheme for reverse pulse voltammetry is very similar to normal pulse voltammetry. The difference relates to the potential outside the pulse, and the direction the pulse is applied. While in normal pulse voltammetry, the potential outside the pulse is such that no electron transfer occurs, in reverse pulse voltammetry, it is designed such that current is at its maximum. In addition, in reverse pulse voltammetry, the applied pulse reduces the current flow, not increases it like in normal pulse voltammetry.

The data that can be obtained from this method is quite similar to normal pulse voltammetry for the system O + ne- ⇌ R. As such, applications for the system O + ne- ⇌ R will not be discussed here, as normal pulse voltammetry is more appropriate.

Characterising the Stability of Products of Electrolysis

The mathematics of a reversible system for this process is well defined. However, for the reaction O + ne- ⇌ R, with R initially absent, any instability in R during the rest pulse effects the result. As such, this method may be used to study the stability of R.

Differential Pulse Voltammetry¹

The principle purpose of differential pulse voltammetry is to increase the sensitivity of normal pulse voltammetry. The differences between it and pulse voltammetry are:

• The base potential increase in small increments with each drop
• The pulse height is maintained at between 10-100 mV above the base potential

In addition, two currents are recorded, one before the pulse and one just before the end of the pulse (where the mercury drop is dislodged). The difference (δi) between the two recorded currents is plotted verses the base potential. Normally the rest period is 0.5 - 4 s, and the pulse width is approximately 50 ms.

The results differ substantially from reverse pulse voltammetry and normal pulse voltammetry, and have a peak as opposed to a monotonic increase. The initial base potential can be interpreted as making a new apparent bulk concentration, different from what exists before. As the pulse height is decreased, the result tends towards the differential of the result of normal pulse voltammetry, giving a qualitative view of the results.

The technique can be applied to non-polarographic electrodes. The base potential establishes a new environment, into which the pulse is applied. This base potential serves to refresh the diffusion properties of the system.

Squarewave Pulse Voltammetry¹

Squarewave voltammetry is a method which combines the sensitivity of pulse voltammetry, and the ability to test products directly that cyclic voltammetry has. However, it lacks the easy qualitative information of the prior methods.

This method starts at a potential, and is pulsed negatively then positively by a potential (∆E_p>), before repeating a positive and negative pulse around a new potential which adds a step (E_step). This means the potentials progress as follows:

Unlike in other pulse voltammetry methods, there is no renewal of the solution from either dropping mercury or being left at a rest potential. The current is usually recorded towards the end of each pulse, and the three different values plotted. These values are the forward current (where ∆E_p> is added), the reverse current (where ∆E_p> is subtracted), and their difference is plotted. The pulse length tends to be 1 – 500 ms, the pulse height 50/n mV, and the step height 10/n mV.

Calculation of the Polarographic Half Wave Potential

For a reversible system O + ne^- ⇌ R where R is initially absent the peak current the difference current reaches a peak at the polagraphic half wave potential, where:

Calculation of Other Electrochemical Parameters

The current recording by this method can be fit to determine the electrochemical parameters of a system to a great deal of accuracy.

Additional Methods

---

Rotated Electrode Voltammetry¹

Electrodes can be made to rotate, and this changes both the way compounds diffuse and the current recorded. The main advantage is that a steady state is reached quickly, and that the rate of mass transfer is quicker. The quicker mass transfer makes currents from some electronic processes larger. In addition, the rate of stirring can be controlled to affect the electronic processes.

Steady state current measurements also have the advantage of allowing charging currents to be ignored. The main electrode used for these techniques is the rotated disk electrode, for which mathematical solutions to the diffusion equations may be calculated. Rotated disk electrodes can be used in pulse voltammetry methods, or linear sweep methods. The results gained are different however, corresponding to the differing diffusion properties, so follow different equations.

Stripping Voltammetry¹

In stripping voltammetry, the concentration of an electroactive species is increased at an electrode, prior to analysis. This method allows for the study of electroactive species in highly dilute solution (10^-10 – 10^-11 M). The most common method of concentrating the electroactive species is to have an electrode which incorporates the oxidised or reduced species not in solution.

In addition, the compound may favourably deposit onto the electrode. The electrode is set to a potential so that electrolysis happens, and the oxidised/reduced species build up onto the electrode. The species is then "stripped" (i.e. redissolved) and analysed, most commonly by linear sweep voltammetry or differential pulse voltammetry.

If the species is reduced prior to the method, and oxidised as it is released, it may be referred to as anodic stripping voltammetry. Alternatively, if it is reduced then oxidised, it is cathodic stripping voltammetry. Some species adsorb on the electrode, without requiring electrolysis, and this is referred to as adsorptive stripping voltammetry. (Note: because the diffusion characteristics of the electrodes change due to the absorbed species, the equations used for previous methods do not fully apply.)

Ultramicroelectrodes¹

In all experiments which do not require mercury dropping, an ultramicroelectrode can be used. An ultramicroelectrode is characterised as having an electrode area of smaller than the diffusion layer generated during an experiment. No precise definition of this area exists, but one source functionally defines the electrode as having 1 dimension smaller than 25 μm.¹

At very small electrode sizes, the diffusion to an electrode becomes independent of time, and as such steady state potentials are obtained. The steady state diffusion means that linear diffusion cannot be assumed, which changes the equations listed in other sections.

Ultramicroelectrodes have the advantage of being able to operate at much shorter timescales than standard electrodes. This is because the electrodes take time to respond when the applied potential is changed. The potential of the electrode approaches the applied potential, via a decay with time constant of the "cell time constant". It takes approximately 5 cell time constants to reach the desired potential. The cell time constant is minimised by reducing the electrode size. In addition to allowing measuring at shorter time scales in pulse voltametric methods, faster scan rates are also possible in cyclic voltammetry.

A further advantage of ultramicroelectrodes is that they minimise the potential expended on conducting charges in solution. As such, errors from this effect are minimised, even in poorly conducting solutions.

Electrode Impedance Spectroscopy¹

Small amplitude oscillating potentials may be applied to solutions of electroactive species to study their properties. These methods have the advantage over traditional methods due to increased precision. Small amplitude oscillations increase precision over measurement where large changes are made continually due to the ability of average results over long periods of time.

In addition, the treatment of diffusion is usually simplified, and the range of frequencies which can be studied is large. What is measured is the impedance (i.e. the effective resistance and capacitance at the frequency used) of the cell at various frequencies. In electrode impedance spectroscopy, both oxidised and reduced forms of the redox couple must be present. The potential to which the oscillation is applied is the equilibrium potential.

The value which this method is interested in is the "faradic impedance" i.e. the impedance from the oxidation and reduction at the electrode surface. The faradic impedance must be separated from the impedance corresponding to the solution resistance, and the electrode charging. The faradic impedance can be obtained by subtracting the impedance with no electroactive species present. However, the faradic impedance is more commonly obtained from variation in capacitance and resistance with oscillator frequency.¹

Alternating Current Polarography¹

An AC current can be combined with a pulse in voltage as in pulse voltametric methods. In such a method, the alternating current occurs at a much faster rate (usually oscillating at the rate 10¹–10⁵ Hz) than the pulse, and to a much lower magnitude. Between pulses, the diffusion layer is renewed, either stirring or by a mercury drop dropping. The method works by the pulse setting up the ratio of oxidised and reduced compounds desired, and the AC pulse measuring them. As such, it is common to set up the system with only one of the oxidised or reduced forms.

Alternating Current Voltammetry¹

An alternating current (AC) pulse can also be combined with linear sweep(s) to create alternating current voltammetry. The AC pulse must be much faster than the sweep rate for this technique and is usually in the range 10¹–10⁵ Hz. Similar to alternating current polarography, the aim of the sweeping potential is to change the concentration of compounds local to the electrode.

The maths of this system is largely very similar to alternating current polarography, and hence its applications are also similar. In fact, if the electrochemical process is reversible at the scan rate of the linear sweep, all of the applications listed in the alternating current polarography section apply.

Potentiostat

Browse now

Keep Reading...

---

Related Products

---